Thirty-one tone equal temperament, also called 31-tET, 31-EDO, 31-et, or tricesimoprimal meantone temperament, is the scale derived by dividing the octave into 31 equally large steps. The term ‘Tricesimoprimal’ was first used by Adriaan Fokker. Each step is equivalent to a frequency ratio of the 31st root of 2, or 38.71 cents. 31’s perfect fifth is flat of the just interval 3:2 (over five cents), as befits a tuning supporting meantone, but the major third is less than a cent sharp (of just 5:4). 31’s approximation of 7:4, a cent flat, is also very close to just. Because of these near-just values 31-et is relatively quite accurate and is in fact the sixth zeta integral edo. Many 7-limit JI scales are well-approximated in 31 (with tempering, of course). It also deals with the 11-limit fairly well, and is consistent through it, but is the optimal patent val for the rank five temperament tempering out the 13-limit comma 66/65. It also provides the optimal patent val for mohajira, squares and casablanca in the 11-limit and huygens/meantone, squares, winston, lupercalia and nightengale in the 13-limit.
Linear Temperaments
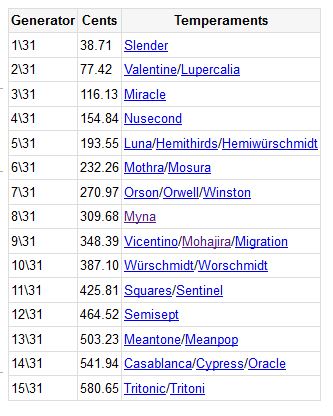
1\31 octave – approx. 38.71¢ – Diesis or up-unison
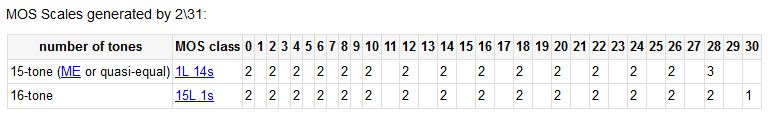
A single step of 31-edo is about 38.71¢. Intervals around this size are called dieses (singular ‘diesis’). In 31 it is equivalent to the difference between one octave and three stacked major thirds (C to E, to G#, to B#, but B# ≠ C), or four minor thirds (C to Eb to Gb to Bbb to Dbb ≠ C). In the 11-limit, the diesis stands in for just ratios 56:55 (31.19); 55:54 (31.77¢); 49:48 (39.70¢); 45:44 (38.91¢); 36:35 (48.77¢); 33:32 (53.27¢) and others. The diesis is a defining sound of 31edo; when it does not appear directly in a scale, it often shows up as the difference between two or more intervals of a similar size
2\31 octave – approx. 77.42¢ – Minor Semitone or Chromatic Semitone or Small Minor Second or downminor 2nd
The difference between a major and minor third. The more ‘expressive’ of the ‘half steps,’ and the larger of 31’s two “microtones”. In meantone, it is the chromatic semitone, the interval that distinguishes major and minor intervals of the same generic interval class (eg. thirds). 2\31 stands in for just ratios 28:27 (62.96¢); 25:24 (70.67¢); 22:21 (80.54¢); 21:20 (84.45¢) and others
MOS Scales generated by 2\31:
3\31 octave – approx. 116.13¢- Major Semitone or Diatonic Semitone or Large Minor Second or minor 2nd
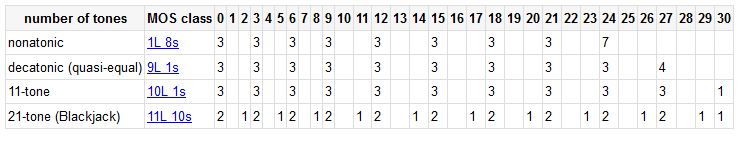
The larger and clunkier of the 31edo semitones. In meantone, it is the diatonic semitone which appears in the diatonic scale between, for instance, the major third and perfect fourth, and the major seventh and octave. 3\31 stands in for just ratios 16:15 (111.73¢); 15:14 (119.44¢) and others. It is notable that two of these make an 8/7; this implies that the 3\31 is a secor and generates miracle temperament.
MOS Scales generated by 3\31:
4\31 octave – approx. 154.84¢ – Neutral Tone or Neutral Second or mid 2nd
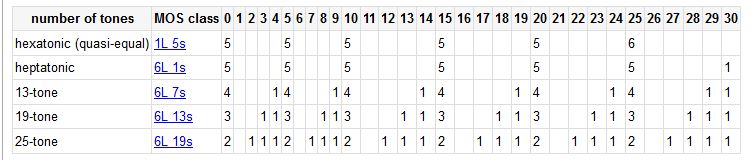
Exactly one half of the minor third and twice the minor semitone. 4\31 stands in for 12:11 (150.64¢); 35:32 (155.14¢); 11:10 (165.00¢) and others. Although neutral seconds are typically associated with the 11-limit, 4\31 approximates the 7-limit interval 35/32 quite well, as the 5th harmonic of the 7th harmonic or vice versa, both of which are closely approximated in 31edo. And although 31 is not extremely accurate in the 11-limit, it is notable that since 11 and 3 are both flat, the interval that distinguishes them (12/11) is only about 4.5¢ off. Generates nusecond temperament.
MOS Scales generated by 4\31:

5\31 octave – approx. 193.55¢ – Whole Tone or Major Second or major 2nd
A rather smallish whole tone. Sometimes called melodically dull. As it falls between (and functions as) just whole tones 9:8 and 10:9, 5\31 is considered a “meantone”. Two meantones make a near-just major third. Perhaps it is worth noting that its relative narrowness (to JI 9/8) makes it easier to distinguish from the 8/7 approximation. And although it is over 10¢ flat of 9/8, 5\31 can function as a somewhat “active” (as opposed to perfectly stable) harmonic ninth, and it can be effective in combination with the also-narrow 11th harmonic. Indeed, the 11/9 approximation is excellent. Try, for instance 31’s version of a 4:6:9:11 chord (steps 0-18-36-45).
MOS Scales generated by 5\31:
6\31 octave – approx. 232.26¢ – Supermajor Second or upmajor 2nd
Exactly one half of a narrow fourth, twice a major semitone, or thrice a minor semitone. In 7-limit tonal music, 6\31 closely represents 8:7 (231.17¢). In meantone, it is a diminished third, eg. C to Ebb. Generates mothra temperament.
MOS Scales generated by 6\31:
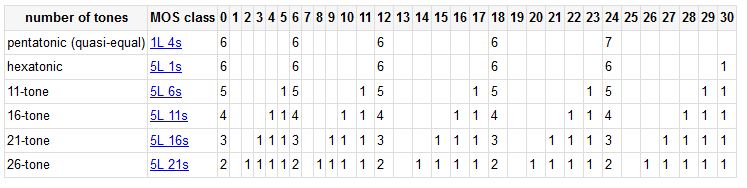
7\31 octave – approx. 270.97¢ – Subminor Third or downminor 3rd
Exactly one half of a superfourth (11:8 approximation). In 7-limit tonal music, 7\31 stands in for 7:6 (266.87¢). In meantone temperament, it is an augmented 2nd, eg. C to D#. Generates orwell temperament.
MOS Scales generated by 7\31:
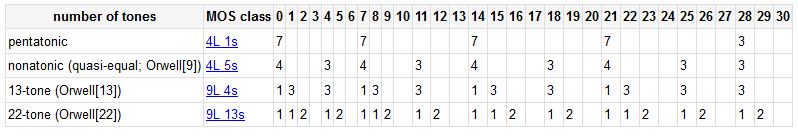
8\31 octave – approx. 309.68¢ – Minor Third
A minor third, closer to the just 6:5 (315.64¢) than 12-edo, but still on the flat side. Exactly twice a neutral second, four times a minor semitone, and half of a large tritone. Generates myna temperament.
MOS Scales generated by 8\31:
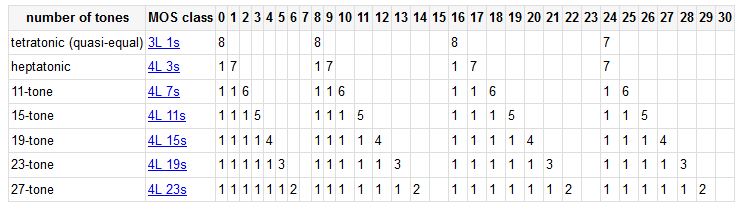
9\31 octave – approx. 348.39¢ – Neutral Third or mid 3rd
A neutral 3rd, about 1¢ away from 11:9 (347.41¢). 9\31 is half a perfect fifth (making it a suitable generator for mohajira temperament), and also thrice a major semitone. It is closer in quality to a minor third than a major third, but indeed, it is distinct. It is 11¢ shy of 16/13 (359.47¢), suggesting a 13-limit interpretation for 31edo. However, its close proximity to 11/9 makes it hard to hear it as 16/13, which in JI has a different quality (and, as a neutral third, is more “major-like” than “minor-like”). Also, its inversion, 22\31 (851.61¢) is wide of the 13th harmonic by about 11¢, which leaves the 143rd harmonic only about 2¢ wide after cancelling with the narrow 11th harmonic, while all the lower harmonics are either near-just or narrow. This means the errors can accumulate, for instance, with 13/9 (636.62¢) represented by 17\31 (658.06¢), a good 21.4¢ sharp.
MOS Scales generated by 9\31:

10\31 octave – approx. 387.10¢ – Major Third
A near-just major 3rd (compare with 5:4 = 386.31¢). Has led to the characterization of 31-edo as “smooth”. Generates wurshmidt/worshmidt temperaments.
MOS Scales generated by 10\31:
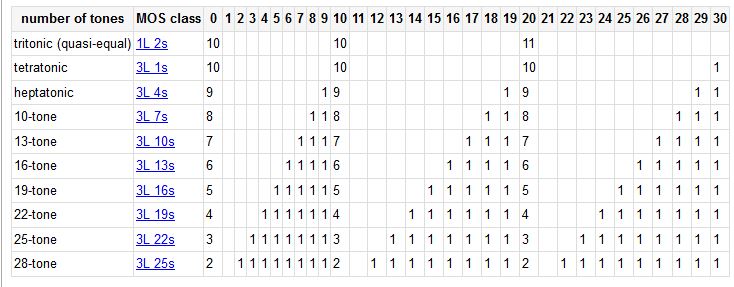
11\31 octave – approx. 425.806¢ – Supermajor Third or upmajor 3rd
11\31 functions as 14:11 (417.51¢), 23:18 (424.36¢), 32:25 (427.37¢), 9:7 (435.08¢) and others. In meantone temperament, it is a diminished fourth, eg. C to Fb. It is notable as closely approximating an interval of the 23-limit, suggesting the possibility of treating 16\31 (619.35¢) as a flat version of 23/16 (628.27¢). It is perhaps also notable for being close to 6\17, the bright major third of the ever-popular 17edo. Generates squares temperament.
MOS Scales generated by 11\31:
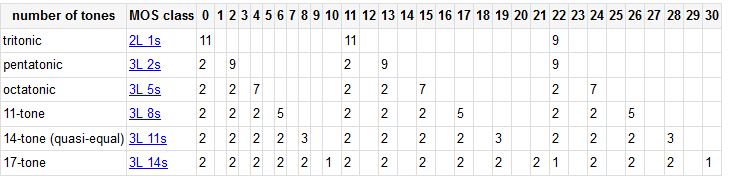
12\31 octave – approx. 464.52¢ – Narrow Fourth or Subfourth or down 4th
Exactly twice a supermajor second, thrice a neutral second, or four times a minor second. In the 7-limit, 12\31 functions as 21:16 (470.78¢). It is also quite close to the 17-limit interval 17/13 (464.43¢), although 31edo does not offer up reasonable approximations of the 17th or 13th harmonics to help make this identity clear. This interval and its inversion 19\31 (735.48¢, a superfifth) are notable for being the only intervals in the 31edo octave larger than the 3\31 diatonic semitone (and smaller than its inversion, 28\31) that are not 11-limit consonances, and the only intervals in the 31edo octave that are not 15-limit consonances. Generates semisept temperament.
MOS Scales generated by 12\31:
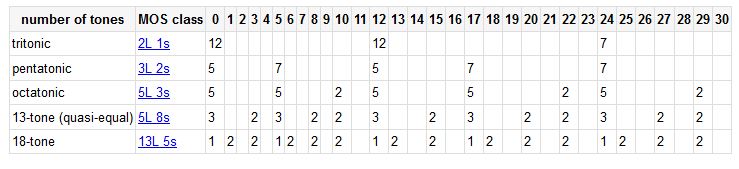
13\31 octave – approx. 503.23¢ – Perfect Fourth
A slightly wide perfect fourth (compare to 4:3 = 498.04¢). As such, it functions marvelously as a generator for meantone temperament.
MOS Scales generated by 13\31:
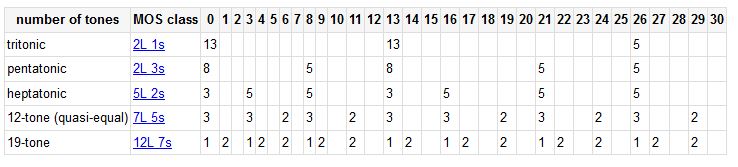
14\31 octave – approx. 541.94¢ – Superfourth or up 4th
Exactly twice a subminor third. Functions as both the 11:8 (551.32¢) and 15:11 (536.95¢) undecimal superfourths (121/120 is tempered out). Thus it makes possible a symmetrical tempered version of an 8:11:15 triad. As 11/8, 14\31 is about 9¢ flat; however, it fits nicely with the also-flat 9/8, allowing a near-just 11/9. Nonetheless, most 11-limit chords in 31edo have a somewhat unstable quality which distinguishes them from their just counterparts. Generates casablanca temperament.
MOS Scales generated by 14\31:
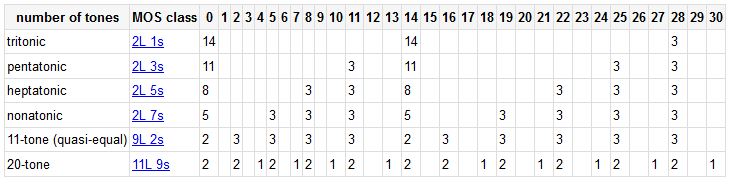
15\31 octave – approx. 580.65¢ – Small Tritone or Augmented 4th or Subdiminished Fifth or downdim 5th
In 7-limit tonal music, functions quite well as 7:5 (582.51¢). Exactly thrice a whole tone. Generates tritonic temperament.
MOS Scales generated by 15\31:
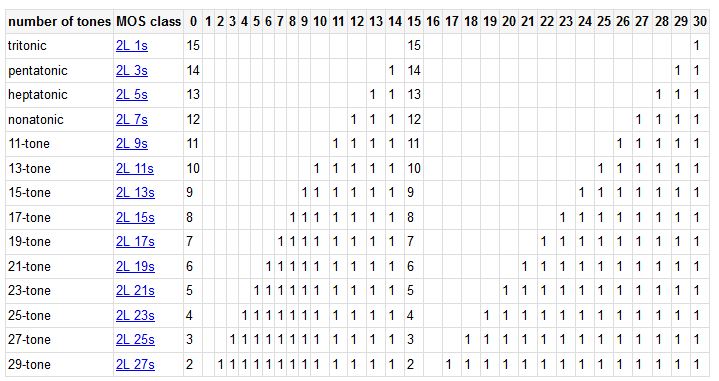
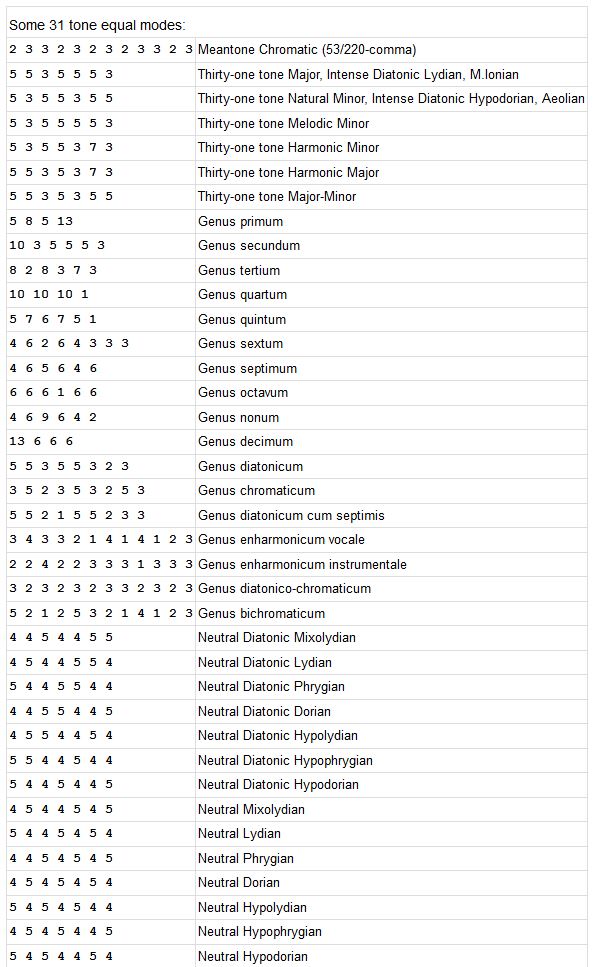
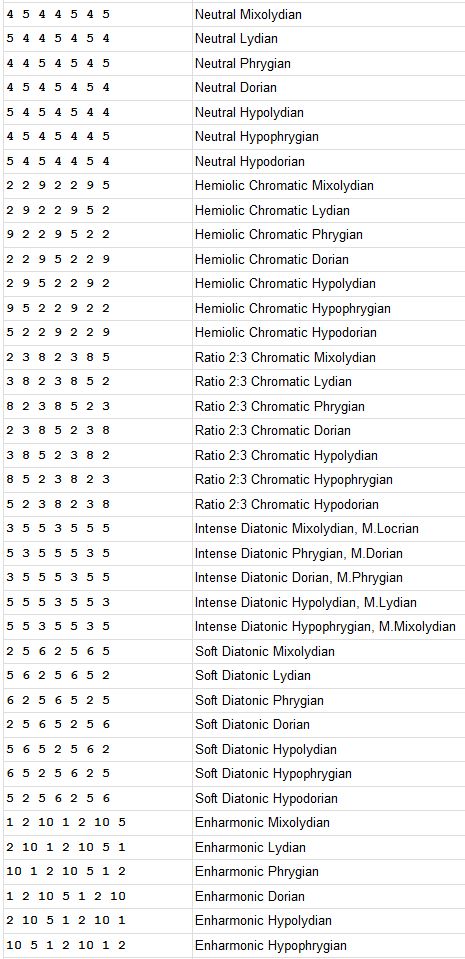
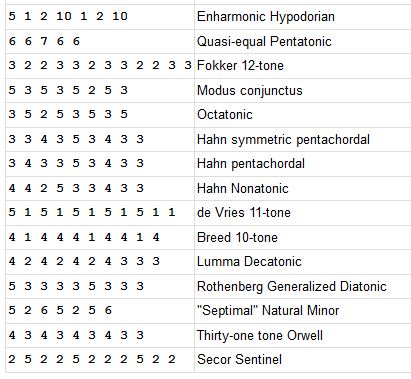
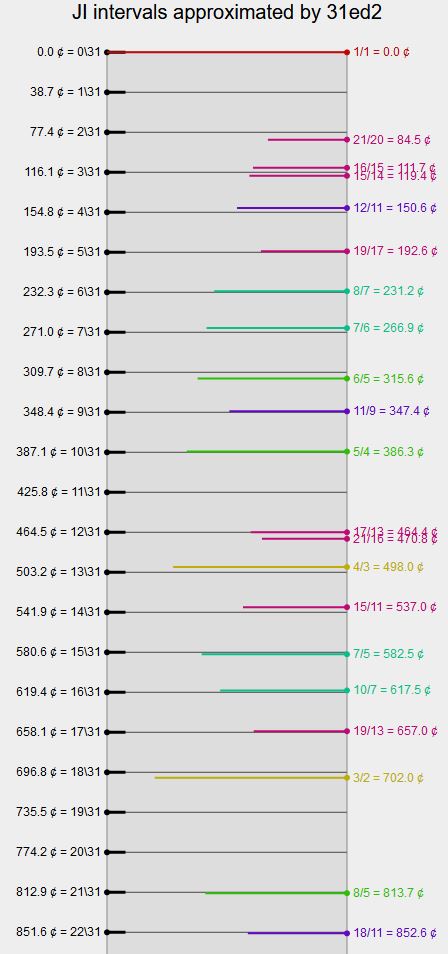
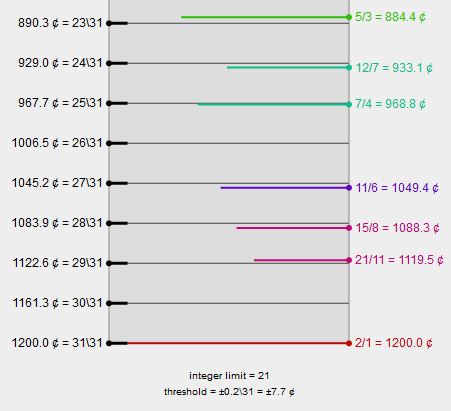
Taken from the xenharmonic wiki to preserve some knowledge